Curated and Reviewed by
Lesson Planet
This The Volume Formula of a Sphere lesson plan also includes:
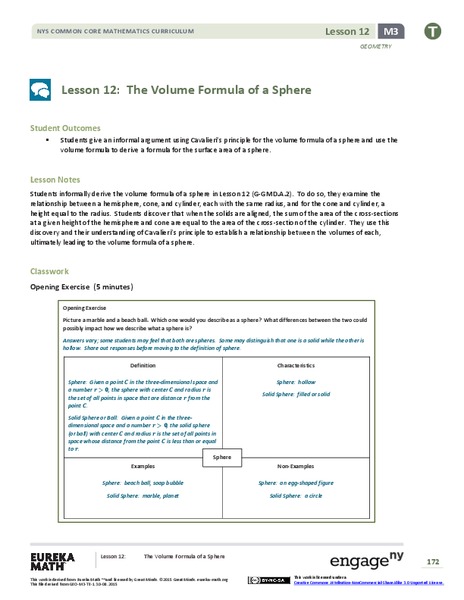
What is the relationship between a hemisphere, a cone, and a cylinder? Using Cavalieri's Principle, the class determines that the sum of the volume of a hemisphere and a cone with the same radius and height equals the volume of a cylinder with equal radius and height. The lesson continues to incorporate the volume formula to derive the formula for the surface area of a sphere.
26 Views
18 Downloads
CCSS:
Designed
Concepts
Additional Tags
Instructional Ideas
- Research and find visuals of Cavalieri's Principle proof of the volume of a sphere that can be projected to show the class during the discussion
- It may be beneficial to split the lesson into two days, one day for the volume and one day for the surface area
Classroom Considerations
- The concept may be difficult to grasp without being able to see it; add visuals to make the lesson more understandable
- The twelfth lesson in a 14-part series
Pros
- Includes a graphic organizer that is commonly used with vocabulary
- Provides some images that will be helpful to those struggling to envision what is being discussed
Cons
- None